Lecture 4
Today
Response functions of a noninteracting system.
Lindhard function and its structure.
Reading
1. Non-interacting system
The observables
Then
Noting the identity
We find
In the frequency domain
where
The convergence factor
so that
Next, we consider the density response function. The density operator is
so
Think of
So for the density response commensurate with the external fields
This means
So that
This is the so-called Lindhard function.
Digression on spin response
When spin is considered, we need to consider both spin densities
Then the spin-resolved density–density response function is defined as
Clearly,
What we call the longitudinal spin response:
A conclusion that one can draw immediately is that the spin–density response functions vanish in the paramagnetic state due to the obvious additional symmetry
For a non-interacting electron gas, we have
Oftentimes, we need to describe systems or excitations for which spin is no longer a good quantum number. For this purpose we need the spin density (in units of
where
which have following properties
Additionally
So
Then we may define the transverse spin-spin response function
2. The Lindhard function
The Lindhard function in Eq.
Now we separate the above into two sums over the occupied states
where the second term comes from the replacement
Using the dispersion relation for a free-electron gas
where
Now define a function
which is antisymmetric:
We find
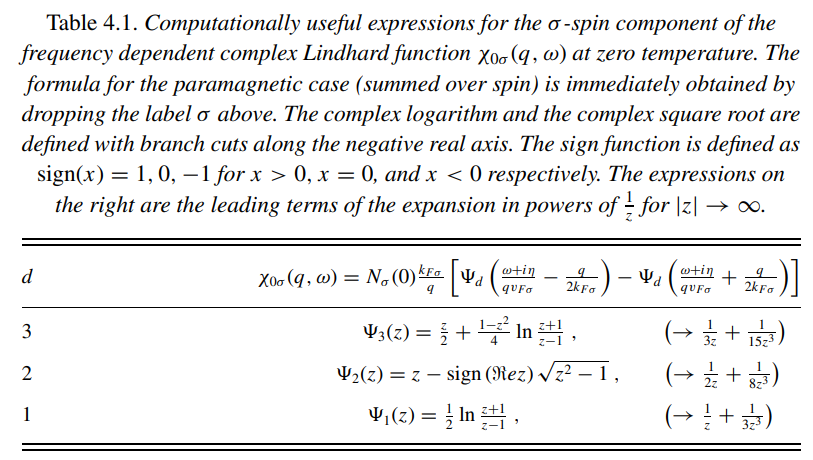
The static limit
The most important feature in the static Lindhard function is the singularity at
with
In the long-wavelength limit
This result is totally reasonable since the susceptibility is directly related to the phase space volume that allows for zero-energy excitation, which should be none but the number of states on the Fermi level in the
Finite frequencies
Rather general insight can be gained by looking at the Lindhard function, even though it characterizes the density response in an ideal electron gas. The most revealing feature of the Lindhard function lies in its imaginary part, the spectral function, which is related to the density structure factor. The spectral function of a 3-dimensional electron gas at
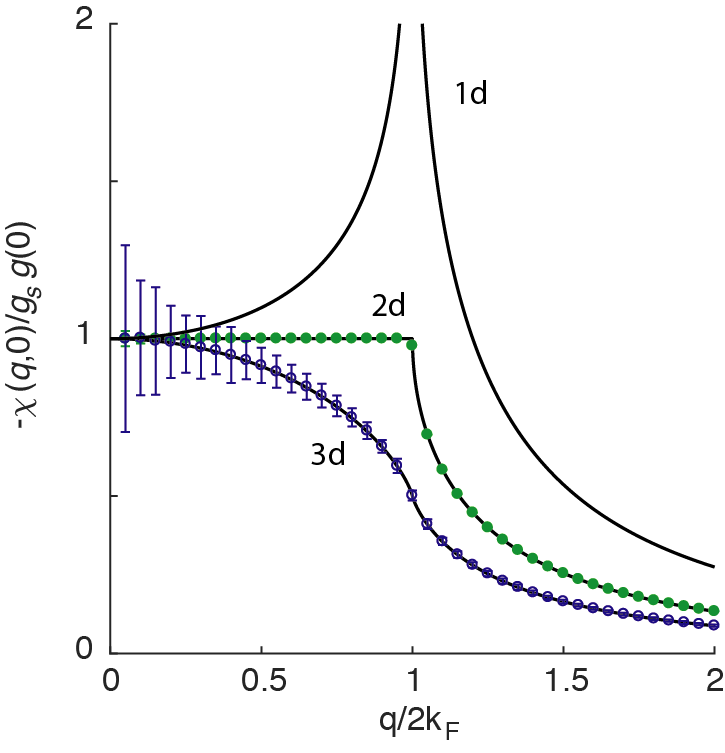
For the zero temperature spectral function, its imaginary part looks like
The integrand is non-zero only if (1)
For a given
If
For
For all values of
Inspecting
Clearly, for
Notes and references