Lecture 19
04/27/23, Th.
Today
Kane-Mele model of 2D TI
Topological insulator and semimetals
Integer quantum Hall effect
1. Kane-Mele model of 2D TI
Consider two copies the Haldane models, which are time-reversed images of each other. As the Berry curvature or flux changes sign under time reversal,
So the combined system has no berry flux through the Brillouin zone. But there will be a pair of degenerate edge states that are Kramer's pair of each other.
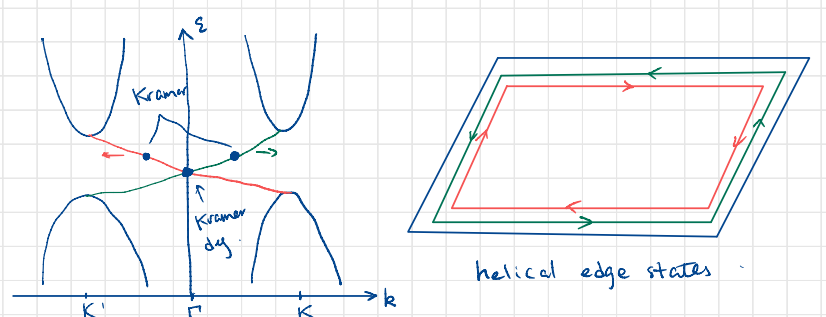
Kramers' degeneracy comes from time-reversal symmetry. For spin-1/2, the time-reversal operation is
where
which is a Bloch function transforms as the irreducible representation (不可约表示) of
If the Hamiltonian has time-reversal symmetry, this means
Therefore,
This is precisely what the Kane-Mele model does, except the staggered magnetic flux is replaced by spin-orbit coupling. To write down the Kane-Mele model, we need three sets of Pauli matrices
So the low-energy effective Hamiltonian is
So the bulk spectrum is two-fold degenerate within each valley
Initially, Kane and Mele thought there will be a quantized spin current, so they give the name quantum spin Hall effect, describable by a spin Chern number
But the problem is if spin is not good quantum number (with symmetry being broken ever so slightly), so it cannot be a conserved quantity and the quantization gets destroyed.
It turns out, what is important is the Kramer's degeneracy protected by TR symmetry. As edge states
It is argued that even with strong disorder, the reflected amplitude must be odd under time-reversal symmetry, so it should be zero. The consequence is that the helical edge states are immune to strong disorder and Anderson localization. From the Landauer formula, we have
The helical edge states protected by the time-reversal symmetry. But what is the bulk topological invariant? The exact formulation is algebraically involved. We will get a first idea with a pictorial argument.
Consider the edge states. We note that if
where
In the case of a 2D insulator, its edge Brillouin zone has two TRIMS:

The case on the left is considered trivial, because it can be continuously deformed into an insulator, by changing the chemical potential for example. The case on the right is the topological. So from the edge state point of view, the topology protected by time reversal symmetry has two distinct classes, entailing a
In bulk
where
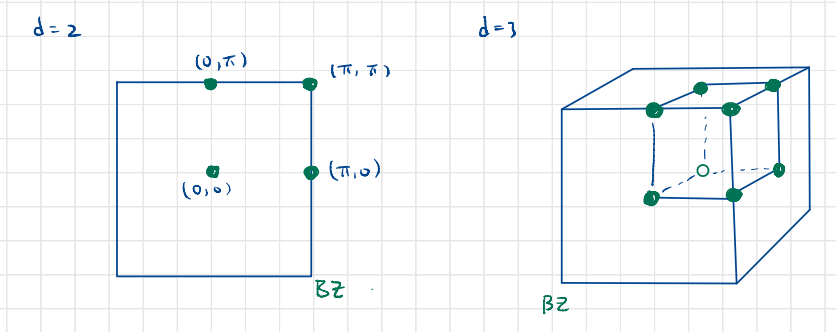
If
2. Weyl semimetal
A minimal model for a Weyl semimetal can be written again in a two-band Hamiltonian.
where
Here
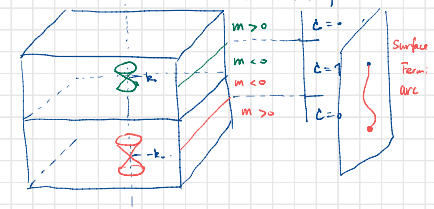
The 2D Brillouin zone with
I will stop here our discussion on these topics in view of time, and switch gear to the more traditional topic of another topological system.
3. Integer quantum Hall effect
3.1 Phenomenology
The quantum Hall effect is typically induced in a 2-dimensional electron gas (2DEG) by a perpendicular magnetic field.
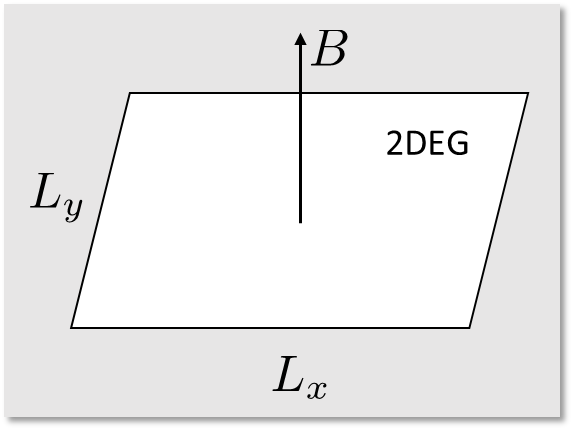
It is called quantum Hall effect because the Hall conductivity is quantized
where the filling factor
In a typical Hall measurement, we have current flowing in the longitudinal direction, with the transverse current to be zero (open circuit).
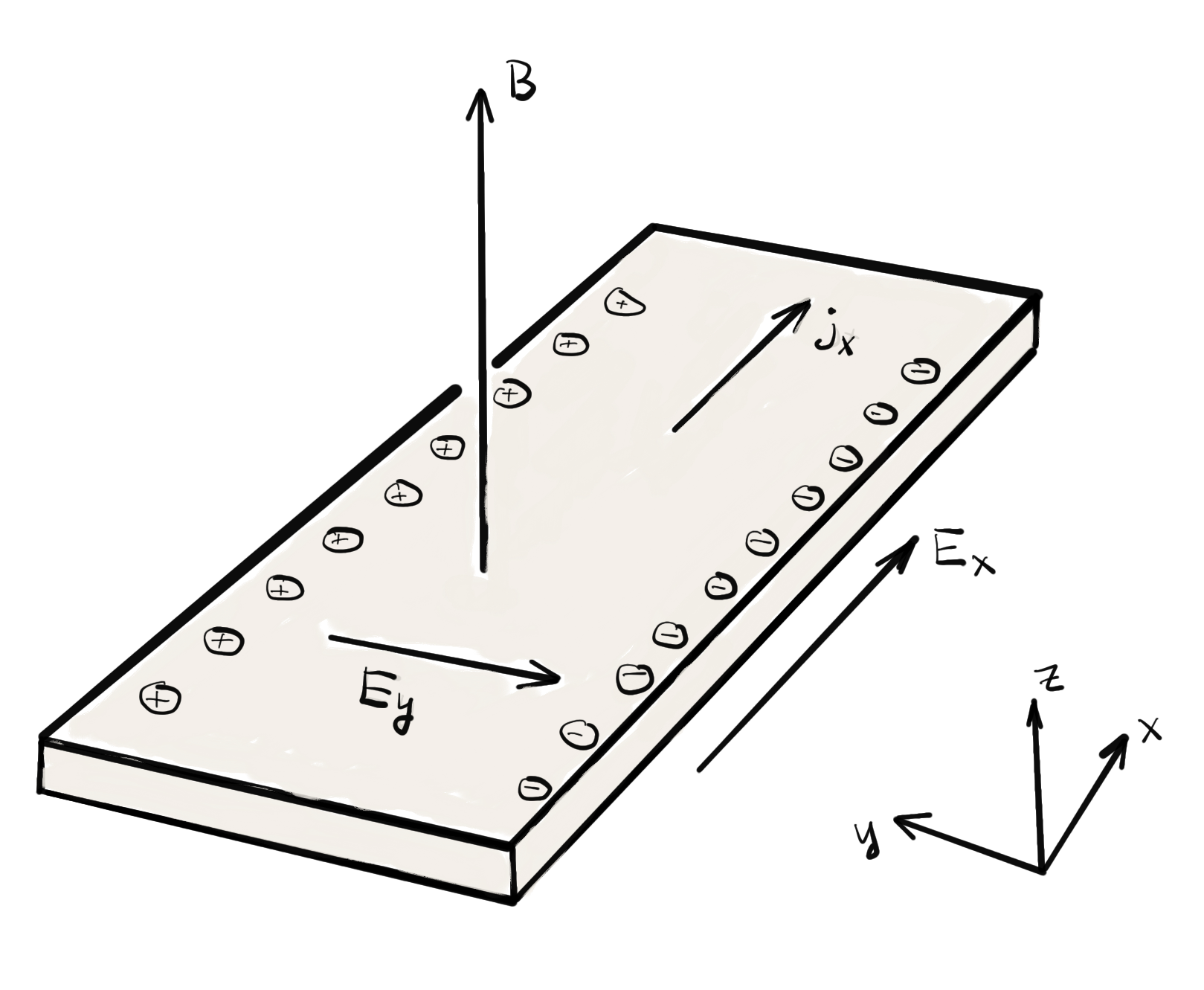
In Drude theory
In steady state
where the cyclotron frequency is
Setting
That is
Note the Hall resistivity is independent of scattering time.
In the quantum Hall measurement, the Hall resistance
so the filling factor
the number of electrons per flux quantum threading the system.
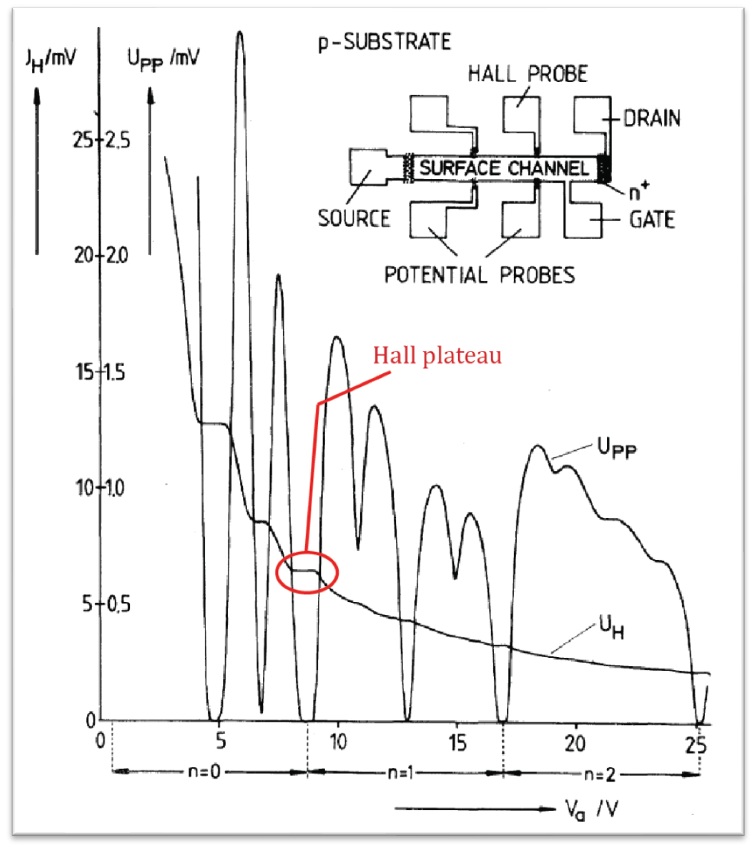

At the Hall plateau
there is precice quantizatoin of
The question is: why the precise quantization? By now, you probably have guessed it is the same thing as the quantum anomalous Hall effect. And you are right. But let's see how this can be understood.
3.2 Disorder is necessary
A curious thing about the integer quantum Hall effect is that disorder is important! Let's consider a perfect 2DEG that is translationally and rotationally invariant. In the lab frame
There is no current.
Now in a moving frame with a constant velocity
and the electric and magnetic fields of the Lorentz boost is
such that
There can be no quantization! As the argument only involves density, it applies whether the theory is quantum or classical.
To have quantization, we must break the Lorentz covariance. We need inhomogeneity (disorder) to break the translational or rotational invariance.
3.3 Landau levels
Choosing the Landau gauge for
the single-electron Hamiltonian reads
which is translationally invariant in
where
So
which is highly degenerate as it is

We use
where
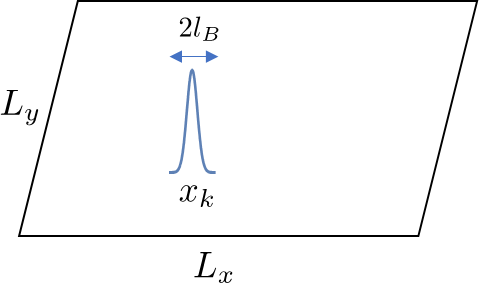
What exactly is the degeneracy of a Landau level? We need
States on the left and right edges can have vastly different k values. Noting
Thus, there there is one state per flux quantum in an LL. So when
Consider a wavepacket and its time evolution is clearly
Now consider
so the motion is clearly periodic, like the classical cyclotron orbit motion.
3.4 Current response
Now we want to calculate the current response of a quantum Hall system. We will restrict ourselves to the lowest Landan level (LLL)
The current density for a given
So the current in y-direction is
Here, the canonical momentum in y-direction
There is no current in the ground state, for sure. This is expected since
But, this independence of energy on
which also preserves translation invariance in

The Hamiltonian can be re-written
where we have new guiding center
and introduced a drift velocity
So the LL energies become
So the current becomes
So the current density in y direction is
So
So the filled LLL has a quantized Hall conductivity.
We will then try and understand the topological nature of the quantized Hall effect, by figuring out the bulk topological invariant and the corresponding edge states.